Addressing the five ‘big questions’ in problem-solving with NRICH

Addressing the five ‘big questions’ in problem-solving with NRICH
The importance of ensuring learners acquire the problem-solving skills which will enable them to thrive both socially and economically in their increasingly automated world is widely recognised (Luckin et al., 2017). Nevertheless, government inspectors have reported serious concerns about the quality and quantity of problem-solving in our schools (Ofsted, 2015). This summer schools were challenged to reflect on ‘Five big questions for problem-solving’ (EEF, 2021). In this blog, we will consider each of those five questions and explore the ways that the NRICH team is supporting schools to address them.
Question one: Do teachers in your school select genuine problem-solving tasks for which pupils do not already have a ready-made method available?
Too often, learners are presented with routine word problems which merely require the application of a known algorithm. ‘Genuine’ problems enable them to make their own problem-solving decisions by choosing their own strategies and enabling them to compare their approach with those of other learners, thus developing their problem-solving efficiency and flexibility. At NRICH, our award-winning activities allow learners to develop these key skills alongside the confidence to tackle genuine problems. Moreover, our ‘low threshold, high ceiling‘ approach enables everyone to get started on the problem while ensuring a suitable level of challenge too, making them ideal for whole-class teaching.
Question two: Are pupils given the opportunity to see – through multiple worked examples – to use, and to compare different approaches to solving a problem?
Many problems can be explored in more than one way. Working flexibly, making connections between different areas of the curriculum and reflecting on various problem-solving approaches are key steps towards becoming a more fluent mathematician. NRICH encourages learners to develop these skills in these two ways:
Our primary, secondary and post-16 Live Problems invite learners to explore and submit their ideas to the team. We review each submission that we receive and publish a selection on our website showcasing different approaches and the reasoning behind them.
Our NRICH online activities sometimes feature ‘hide and reveal’ buttons showcasing different starting points towards a solution for learners to explore further for themselves. This approach enables learners to widen their range of strategies for solving unfamiliar problems and develop alternative approaches to explore when they get stuck using their first-choice strategy.
Question three: Are pupils encouraged to use visual representations to support them to solve a problem?
One of the most important approaches towards solving an unfamiliar problem is drawing a good diagram. Learning to draw diagrams is a skill which we encourage learners of all ages to develop alongside their other mathematical skills and knowledge. From sketching graphs to drawing a bar model, good diagrams can help learners clarify their understanding and identify possible ways forward.
Our four steps towards problem-solving feature highlights the importance of drawing a diagram to enable young learners to get started on a problem. We often highlight a useful diagram, table or sketch graph in the solutions chosen for publication. As learners progress through their learning, the team model more specific drawing skills, such as sketching a graph to help solve a STEP problem.
Question four: Are pupils supported to monitor, reflect on, and communicate their reasoning and choice of strategies, possibly through the use of prompt questions?
NRICH encourages learners to reflect on their learning using this approach inspired by the Strands of Mathematical Proficiency model introduced by Kilpatrick et al. (2001).
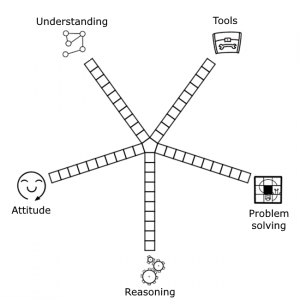
Our approach uses child-friendly language that teachers and parents can share with students five key ingredients that characterise successful mathematicians. At NRICH, we believe that learning mathematics is about much more than just learning topics and routines. Successful mathematicians understand the curriculum content and are fluent in mathematical skills and procedures, but they can also solve problems, explain their thinking and have a positive attitude about themselves as learners of mathematics.
With this in mind, we have created this self assessment tool to help learners recognise where their mathematical strengths and weaknesses lie. We hope learners will explore NRICH activities and then take time to reflect on their own mathematical capabilities using our model.
Question 5: Is professional development time allocated to develop teachers’ pedagogical understanding of problem-solving, with particular support for early career teachers?
NRICH supports teachers to maximise the potential of our activities by offering free, regular professional development for teachers. Each session is delivered online, enabling teachers to access the support wherever they are based, reducing teacher travel and cover costs for schools. We also record the sessions and upload them to our website so that schools can access them for future professional development days or staff/department meetings in their settings.
The live sessions are led by NRICH team members and they link directly to our latest primary, secondary and post-16 Live Problems. This approach enables teachers to consider the possibilities of the activities with the NRICH team before exploring them the next day with their own classes. Later, they are invited to share their classwork with our team for possible publication on the NRICH website.
Summary
The five ‘big questions’ provide excellent starting points for evaluating the teaching and learning of problem-solving in different settings. I hope that this blog shares an insight into the different ways that NRICH can support schools to address the five questions for themselves by engaging with our activities, Live Problems and teacher webinars.
Dr Ems Lord FCCT
Director of NRICH
Centre for Mathematical Sciences
University of Cambridge
Selected references
EEF. (2021). EEF Blog: Integrating evidence into maths teaching – guiding problem-solving. Accessed from https://educationendowmentfoundation.org.uk/news/eef-blog-integrating-evidence-into-mathematics-guiding-problem-solving /
Kilpatrick, J. Swafford, J., & Findell, B. (2001). Adding it up: Helping children learn mathematics (Vol. 2101). J. Kilpatrick, & National research council (Eds.). Washington, DC: National Academy Press.
Luckin, R., Baines, E., Cukurova, M., Holmes, W., & Mann, M. (2017). Solved! Making the case for collaborative problem-solving. Accessed from http://oro.open.ac.uk/50105/1/solved-making-case-collaborative-problem-solving.pdf
Ofsted. (2015). Better Maths Conference Spring Keynote 2015. Accessed here https://www.slideshare.net/Ofstednews/better-mathematics-keynote-spring-2015
